
4.2 Straight-Line Motion: Connecting Position, Velocity, and Acceleration
3 min read•february 15, 2024
Meghan Dwyer
Meghan Dwyer
You might be wondering now that you are a master at calculating all kinds of derivatives under what scenarios you might need this knowledge. One of the many ways we can apply derivatives to real life is through solving rectilinear motion problems involving position, speed, velocity, and acceleration. 🚗
🌊 Derivatives and Motion
How is this possible? Well, remember from previous units that the derivative is used to determine the instantaneous rate of change or the rate of change of a function at a specific point. This means that…
🛥️Velocity and Speed
If we have a function that represents the position of an object as a function of time, , its derivative, , would represent the velocity of the object at a specific point in time. You may also see written as since the definition of velocity is the rate of change of position with respect to time. The instantaneous velocity is always signed.
When velocity is negative, the object is moving to the left: ➖ = ⬅️.
When velocity is positive, the object is moving to the right: ➕ = ➡️.
If we want just the speed, we simply take the magnitude (absolute value) of the velocity.
🚤Acceleration
Similarly, the derivative of a function that represents the velocity of an object describes the acceleration of the object at a specific point in time since acceleration is defined as the rate at which an object changes its velocity. Furthermore, since the first derivative of an object’s position is the object’s velocity and the first derivative of an object’s velocity is the object’s acceleration, we find that the second derivative of a function that represents the position of an object also describes the acceleration of the object at a specific point in time.
If the acceleration is positive that means that the velocity is increasing in the direction of the motion over time, and if the acceleration is negative that means that velocity is decreasing in the direction of the motion over time. And if the acceleration is zero that means that the object is moving at a constant rate.
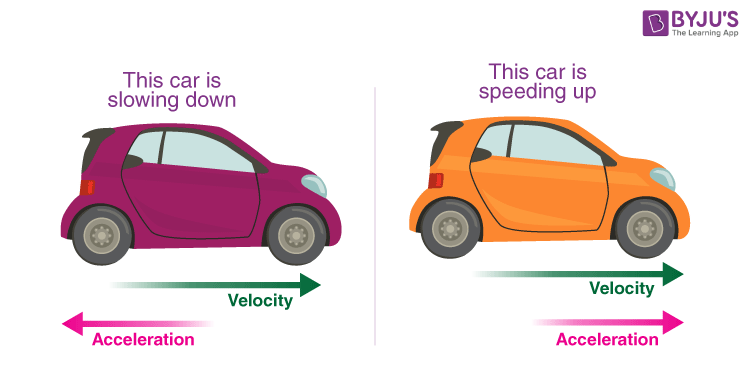
Image Courtesy of BYJU’S
You can also think of it like this:
When , have the same sign, then the particle is speeding up! ➕➕ = ⬆️
When , have different signs, then the particle is slowing down! ➕➖ = ⬇️
📝Rectilinear Motion: Practice
Let’s put this knowledge into practice!
❓Rectilinear Motion: Problems
Question 1:
A particle moves along the x-axis. The function gives the particle’s position at any time .
What is the particle’s acceleration at
Question 2:
A particle moves along the x-axis. The function gives the particle’s position at any time .
What is the particle’s velocity at
✅ Rectilinear Motion: Solutions
Question 1:
Acceleration is the rate of change of velocity, which is the rate of change of position. This means that we need to find the second derivative of the given position function to find the acceleration of the particle at the given specific point in time:
.
The particle's acceleration is constant at 0 for all , so the particle’s acceleration at is 0.
Question 2:
Velocity is the rate of change of position, which means that we need to find the derivative of the given position function to find the velocity of the particle at the given specific point in time:
.
The particle's velocity is for all , so its velocity at is .
Great work!
Key Terms to Review (17)
a(t)
: The term "a(t)" represents the acceleration of an object at a specific time. It measures how quickly the velocity of an object is changing.Absolute Value
: The distance between a number and zero on the number line, always resulting in a non-negative value.Acceleration
: Acceleration refers to the rate at which an object's velocity changes over time. It measures how quickly an object is speeding up, slowing down, or changing direction.Derivatives
: Derivatives are the rates at which quantities change. They measure how a function behaves as its input (x-value) changes.Key Term: a(t) = 0
: Definition: When the acceleration function, a(t), is equal to zero, it means that there is no change in velocity over time. The object is either at rest or moving with constant velocity.Negative Velocity
: Negative velocity refers to motion in the opposite direction of a chosen positive direction. It indicates that an object is moving backwards or in reverse.Position function
: The position function describes the location of an object in relation to a reference point or origin. It shows how an object's position changes over time.Position, Velocity, Acceleration Analysis
: A method used to analyze the motion of objects by examining their position, velocity, and acceleration as functions of time.Positive Velocity
: Positive velocity refers to motion in the chosen positive direction. It indicates that an object is moving forward or in accordance with a specified reference frame.Rates of Change
: Rates of change refer to how a quantity is changing over time or with respect to another variable. It measures the speed at which something is changing.Rectilinear Motion
: Rectilinear motion refers to the movement of an object along a straight line path. It involves changes in position, velocity, and acceleration without any deviation from a straight line.Second Derivative of Position
: The second derivative of position refers to the rate at which an object's velocity is changing over time. It measures how quickly the object is accelerating or decelerating.Speed
: Speed is simply how fast an object moves, without considering its direction. It measures distance covered per unit of time.v'(t)
: The notation "v'(t)" denotes the first derivative of velocity with respect to time. It measures how an object's speed is changing at any given moment.v(t) = 0
: An equation that represents when the velocity of an object is equal to zero at a specific time t.x''(t)
: The second derivative of a function x(t) with respect to time. It represents the rate of change of acceleration over time.x'(t)
: The derivative of a function x(t) with respect to time, denoted as x'(t), represents the rate of change of the function at any given point in time.4.2 Straight-Line Motion: Connecting Position, Velocity, and Acceleration
3 min read•february 15, 2024
Meghan Dwyer
Meghan Dwyer
You might be wondering now that you are a master at calculating all kinds of derivatives under what scenarios you might need this knowledge. One of the many ways we can apply derivatives to real life is through solving rectilinear motion problems involving position, speed, velocity, and acceleration. 🚗
🌊 Derivatives and Motion
How is this possible? Well, remember from previous units that the derivative is used to determine the instantaneous rate of change or the rate of change of a function at a specific point. This means that…
🛥️Velocity and Speed
If we have a function that represents the position of an object as a function of time, , its derivative, , would represent the velocity of the object at a specific point in time. You may also see written as since the definition of velocity is the rate of change of position with respect to time. The instantaneous velocity is always signed.
When velocity is negative, the object is moving to the left: ➖ = ⬅️.
When velocity is positive, the object is moving to the right: ➕ = ➡️.
If we want just the speed, we simply take the magnitude (absolute value) of the velocity.
🚤Acceleration
Similarly, the derivative of a function that represents the velocity of an object describes the acceleration of the object at a specific point in time since acceleration is defined as the rate at which an object changes its velocity. Furthermore, since the first derivative of an object’s position is the object’s velocity and the first derivative of an object’s velocity is the object’s acceleration, we find that the second derivative of a function that represents the position of an object also describes the acceleration of the object at a specific point in time.
If the acceleration is positive that means that the velocity is increasing in the direction of the motion over time, and if the acceleration is negative that means that velocity is decreasing in the direction of the motion over time. And if the acceleration is zero that means that the object is moving at a constant rate.

Image Courtesy of BYJU’S
You can also think of it like this:
When , have the same sign, then the particle is speeding up! ➕➕ = ⬆️
When , have different signs, then the particle is slowing down! ➕➖ = ⬇️
📝Rectilinear Motion: Practice
Let’s put this knowledge into practice!
❓Rectilinear Motion: Problems
Question 1:
A particle moves along the x-axis. The function gives the particle’s position at any time .
What is the particle’s acceleration at
Question 2:
A particle moves along the x-axis. The function gives the particle’s position at any time .
What is the particle’s velocity at
✅ Rectilinear Motion: Solutions
Question 1:
Acceleration is the rate of change of velocity, which is the rate of change of position. This means that we need to find the second derivative of the given position function to find the acceleration of the particle at the given specific point in time:
.
The particle's acceleration is constant at 0 for all , so the particle’s acceleration at is 0.
Question 2:
Velocity is the rate of change of position, which means that we need to find the derivative of the given position function to find the velocity of the particle at the given specific point in time:
.
The particle's velocity is for all , so its velocity at is .
Great work!
Key Terms to Review (17)
a(t)
: The term "a(t)" represents the acceleration of an object at a specific time. It measures how quickly the velocity of an object is changing.Absolute Value
: The distance between a number and zero on the number line, always resulting in a non-negative value.Acceleration
: Acceleration refers to the rate at which an object's velocity changes over time. It measures how quickly an object is speeding up, slowing down, or changing direction.Derivatives
: Derivatives are the rates at which quantities change. They measure how a function behaves as its input (x-value) changes.Key Term: a(t) = 0
: Definition: When the acceleration function, a(t), is equal to zero, it means that there is no change in velocity over time. The object is either at rest or moving with constant velocity.Negative Velocity
: Negative velocity refers to motion in the opposite direction of a chosen positive direction. It indicates that an object is moving backwards or in reverse.Position function
: The position function describes the location of an object in relation to a reference point or origin. It shows how an object's position changes over time.Position, Velocity, Acceleration Analysis
: A method used to analyze the motion of objects by examining their position, velocity, and acceleration as functions of time.Positive Velocity
: Positive velocity refers to motion in the chosen positive direction. It indicates that an object is moving forward or in accordance with a specified reference frame.Rates of Change
: Rates of change refer to how a quantity is changing over time or with respect to another variable. It measures the speed at which something is changing.Rectilinear Motion
: Rectilinear motion refers to the movement of an object along a straight line path. It involves changes in position, velocity, and acceleration without any deviation from a straight line.Second Derivative of Position
: The second derivative of position refers to the rate at which an object's velocity is changing over time. It measures how quickly the object is accelerating or decelerating.Speed
: Speed is simply how fast an object moves, without considering its direction. It measures distance covered per unit of time.v'(t)
: The notation "v'(t)" denotes the first derivative of velocity with respect to time. It measures how an object's speed is changing at any given moment.v(t) = 0
: An equation that represents when the velocity of an object is equal to zero at a specific time t.x''(t)
: The second derivative of a function x(t) with respect to time. It represents the rate of change of acceleration over time.x'(t)
: The derivative of a function x(t) with respect to time, denoted as x'(t), represents the rate of change of the function at any given point in time.
Resources
© 2024 Fiveable Inc. All rights reserved.
AP® and SAT® are trademarks registered by the College Board, which is not affiliated with, and does not endorse this website.