6.3 Justifying a Claim Based on a Confidence Interval for a Population Proportion
5 min read•january 3, 2023
Josh Argo
Jed Quiaoit
Josh Argo
Jed Quiaoit
A confidence interval for a population proportion is based on sample data and is used to estimate the likely range of values for the population proportion. The confidence interval is constructed at a specific confidence level, which determines the probability that the interval contains the true population proportion.
Since each confidence interval is based on a random sample of data, the interval will either contain the population proportion or it will not. There is a specified probability, based on the confidence level, that the interval will contain the true population proportion. For example, if the confidence level is 95%, there is a 95% probability that the interval contains the true population proportion. 🫙
Concluding a Confidence Interval
Let's return to our confidence interval that was given before:
We are estimating a 95% confidence interval of what proportion of high school math students pass their class. We were given a sample of 85 students where ~75% of them passed. We calculated a confidence interval for the true population proportion based off of our sample. The interval is given in the calculator output below: 🔢
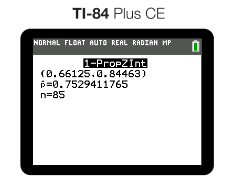
We are given the 95% confidence interval (0.66125, 0.84463) as an estimate of the population proportion of high school students who are passing their math class.
Huh? What the Heck Does This Mean?
In terms of what this means, it means we are 95% confident that the true population proportion of high school students who pass their math class is between 0.66125 and 0.84463. Notice that both of our endpoints are decimals less than 1. This is because we are estimating a proportion, which is always between 0 and 1. Anytime we are calculating any type of proportion, our answer should always be between 0 and 1.
Interpretation Templates
Interpreting a confidence interval for a one-sample proportion should include a reference to the sample taken and details about the population it represents.
"We are C% (90%, 95%, 99%, etc.) confident that the confidence interval for a population proportion captures the population proportion of (CONTEXT)."
Example: For interpreting a 99% confidence interval of (0.268, 0.292), based on the proportion of a nationally representative sample of twelfth-grade students who answered a particular multiple choice question correctly: “We are 99 percent confident that the interval from 0.268 to 0.292 contains the population proportion of all United States twelfth-grade students who would answer this question correctly”
"In repeated random sampling with the same sample size, approximately C% of confidence intervals created will capture the population proportion of (CONTEXT)."

image courtesy of: pixabay.com
The Big Three
When interpreting a confidence interval for a population proportion, there are three things necessary to receive full credit: confidence level, context, and reference to true population proportion. 🧠
(1) Confidence Level
Our confidence level is generally given in the problem. This is the 95%, 90%, 98%, etc. This impacts the z* for our confidence interval and is necessary in including in our interpretation of the interval.
(2) Context
As with anything in AP Stats, context is essential to receive full credit. Anytime we write out an answer, we need to include it in context of the problem being asked. It is no different when interpreting a confidence interval. We need to ask ourselves, "What is this interval estimating?" and include that in our response.
(3) True Population Parameter
We also need to be sure that our answer implies that we are estimating a population proportion, not just a sample proportion. After all, there's no reason to estimate something for our sample because we have the EXACT sample proportion as it was given to us. We are using that sample to estimate the bigger picture with our population. 😊
Testing a Claim Using Intervals
When we are given a population proportion that maybe we don't necessarily believe, we can use a confidence interval based off of a random sample to test that claim. The main way we are going to check the statistical claim is by seeing if the claimed population proportion is within our confidence interval. If it is in our confidence interval, then it is possible that the claim is true. If the claimed value is not in our interval, we may need to investigate further to see if the claim made by an article/study is in fact false. 🔎
Example
In our example above dealing with students passing their math class, let's say that we recently read an article that said only 55% of all US students are passing their math class. Therefore, we took a random sample of 85 US math students and we were given the interval above: (0.66125, 0.84463).
Since 0.55 is not in our interval, we have reason to doubt the article that we read. We should definitely investigate it further. 🕵️
Important Note!
When we are given a claim that we are checking, our expected successes and failures change for our Large Counts Condition that we checked in Unit 6.2. Now that we are given a supposed proportion to be true for the population, we use that to calculate our expected successes and failures. So our large counts condition would change to 0.55(85) ≥ 10 & 0.45(85) ≥ 10, which still holds for this particular problem.
In other words, when we are given an actual p to check this condition, use it. When we aren't given a p-value, use the next best thing by using your p-hat. 🧢
Sample Size, Confidence Interval Width, Confidence Level, and Margin of Error
Confidence Interval Width & Sample Size -- INVERSE relationship: When all other things remain the same, the width of the confidence interval for a population proportion tends to decrease as the sample size increases. For a population proportion, the width of the interval is proportional to 1/sqrt(n). This is because as the sample size increases, the standard deviation of the sampling distribution decreases, which results in a narrower confidence interval.
Confidence Interval Width & Confidence Level -- DIRECT relationship: For a given sample, the width of the confidence interval for a population proportion increases as the confidence level increases. This is because a higher confidence level results in a wider confidence interval, as the interval is constructed using a larger critical value.
Confidence Interval Width & Margin of Error -- 2 x MOE = Width: The width of a confidence interval for a population proportion is exactly twice the margin of error. Recall that width of the interval is calculated by adding and subtracting twice the margin of error to the sample proportion.
🎥 Watch: AP Stats - Inference: Confidence Intervals for Proportions
Key Terms to Review (12)
Confidence Interval
: A confidence interval is a range of values that is likely to contain the true value of a population parameter. It provides an estimate along with a level of confidence about how accurate the estimate is.Critical Value
: A critical value is a specific value that separates the rejection region from the non-rejection region in hypothesis testing. It is compared to the test statistic to determine whether to reject or fail to reject the null hypothesis.Expected Successes and Failures
: Expected successes and failures are calculated probabilities of success and failure based on some underlying model or assumption.Large Counts Condition
: The large counts condition, also known as the "success-failure" condition, is used when applying certain statistical methods to categorical data. It states that for these methods to be valid, both the number of successes and failures must be at least 10.Margin of Error
: The margin of error is a measure of the uncertainty or variability in survey results. It represents the range within which the true population parameter is likely to fall.p-value (in the context of a given proportion)
: The p-value is the probability of obtaining a sample proportion as extreme or more extreme than the observed proportion, assuming that the null hypothesis is true.Random Sample
: A random sample is a subset of individuals selected from a larger population in such a way that every individual has an equal chance of being chosen. It helps to ensure that the sample is representative of the population.Sample Data
: Sample data refers to a subset of observations or measurements taken from a larger population. It is used to make inferences and draw conclusions about the entire population.Sampling Distribution
: A sampling distribution refers to the distribution of a statistic (such as mean, proportion, or difference) calculated from multiple random samples taken from the same population. It provides information about how sample statistics vary from sample to sample.Statistical Claim
: A statistical claim is a statement or hypothesis about a population parameter that can be tested using statistical methods.True Population Proportion
: The true population proportion refers to the actual proportion or percentage of a specific characteristic or attribute within an entire population. It is often estimated using sample data.z*
: In statistics, z* refers to the standardized score obtained from the standard normal distribution. It represents the number of standard deviations a data point is away from the mean.6.3 Justifying a Claim Based on a Confidence Interval for a Population Proportion
5 min read•january 3, 2023
Josh Argo
Jed Quiaoit
Josh Argo
Jed Quiaoit
A confidence interval for a population proportion is based on sample data and is used to estimate the likely range of values for the population proportion. The confidence interval is constructed at a specific confidence level, which determines the probability that the interval contains the true population proportion.
Since each confidence interval is based on a random sample of data, the interval will either contain the population proportion or it will not. There is a specified probability, based on the confidence level, that the interval will contain the true population proportion. For example, if the confidence level is 95%, there is a 95% probability that the interval contains the true population proportion. 🫙
Concluding a Confidence Interval
Let's return to our confidence interval that was given before:
We are estimating a 95% confidence interval of what proportion of high school math students pass their class. We were given a sample of 85 students where ~75% of them passed. We calculated a confidence interval for the true population proportion based off of our sample. The interval is given in the calculator output below: 🔢

We are given the 95% confidence interval (0.66125, 0.84463) as an estimate of the population proportion of high school students who are passing their math class.
Huh? What the Heck Does This Mean?
In terms of what this means, it means we are 95% confident that the true population proportion of high school students who pass their math class is between 0.66125 and 0.84463. Notice that both of our endpoints are decimals less than 1. This is because we are estimating a proportion, which is always between 0 and 1. Anytime we are calculating any type of proportion, our answer should always be between 0 and 1.
Interpretation Templates
Interpreting a confidence interval for a one-sample proportion should include a reference to the sample taken and details about the population it represents.
"We are C% (90%, 95%, 99%, etc.) confident that the confidence interval for a population proportion captures the population proportion of (CONTEXT)."
Example: For interpreting a 99% confidence interval of (0.268, 0.292), based on the proportion of a nationally representative sample of twelfth-grade students who answered a particular multiple choice question correctly: “We are 99 percent confident that the interval from 0.268 to 0.292 contains the population proportion of all United States twelfth-grade students who would answer this question correctly”
"In repeated random sampling with the same sample size, approximately C% of confidence intervals created will capture the population proportion of (CONTEXT)."

image courtesy of: pixabay.com
The Big Three
When interpreting a confidence interval for a population proportion, there are three things necessary to receive full credit: confidence level, context, and reference to true population proportion. 🧠
(1) Confidence Level
Our confidence level is generally given in the problem. This is the 95%, 90%, 98%, etc. This impacts the z* for our confidence interval and is necessary in including in our interpretation of the interval.
(2) Context
As with anything in AP Stats, context is essential to receive full credit. Anytime we write out an answer, we need to include it in context of the problem being asked. It is no different when interpreting a confidence interval. We need to ask ourselves, "What is this interval estimating?" and include that in our response.
(3) True Population Parameter
We also need to be sure that our answer implies that we are estimating a population proportion, not just a sample proportion. After all, there's no reason to estimate something for our sample because we have the EXACT sample proportion as it was given to us. We are using that sample to estimate the bigger picture with our population. 😊
Testing a Claim Using Intervals
When we are given a population proportion that maybe we don't necessarily believe, we can use a confidence interval based off of a random sample to test that claim. The main way we are going to check the statistical claim is by seeing if the claimed population proportion is within our confidence interval. If it is in our confidence interval, then it is possible that the claim is true. If the claimed value is not in our interval, we may need to investigate further to see if the claim made by an article/study is in fact false. 🔎
Example
In our example above dealing with students passing their math class, let's say that we recently read an article that said only 55% of all US students are passing their math class. Therefore, we took a random sample of 85 US math students and we were given the interval above: (0.66125, 0.84463).
Since 0.55 is not in our interval, we have reason to doubt the article that we read. We should definitely investigate it further. 🕵️
Important Note!
When we are given a claim that we are checking, our expected successes and failures change for our Large Counts Condition that we checked in Unit 6.2. Now that we are given a supposed proportion to be true for the population, we use that to calculate our expected successes and failures. So our large counts condition would change to 0.55(85) ≥ 10 & 0.45(85) ≥ 10, which still holds for this particular problem.
In other words, when we are given an actual p to check this condition, use it. When we aren't given a p-value, use the next best thing by using your p-hat. 🧢
Sample Size, Confidence Interval Width, Confidence Level, and Margin of Error
Confidence Interval Width & Sample Size -- INVERSE relationship: When all other things remain the same, the width of the confidence interval for a population proportion tends to decrease as the sample size increases. For a population proportion, the width of the interval is proportional to 1/sqrt(n). This is because as the sample size increases, the standard deviation of the sampling distribution decreases, which results in a narrower confidence interval.
Confidence Interval Width & Confidence Level -- DIRECT relationship: For a given sample, the width of the confidence interval for a population proportion increases as the confidence level increases. This is because a higher confidence level results in a wider confidence interval, as the interval is constructed using a larger critical value.
Confidence Interval Width & Margin of Error -- 2 x MOE = Width: The width of a confidence interval for a population proportion is exactly twice the margin of error. Recall that width of the interval is calculated by adding and subtracting twice the margin of error to the sample proportion.
🎥 Watch: AP Stats - Inference: Confidence Intervals for Proportions
Key Terms to Review (12)
Confidence Interval
: A confidence interval is a range of values that is likely to contain the true value of a population parameter. It provides an estimate along with a level of confidence about how accurate the estimate is.Critical Value
: A critical value is a specific value that separates the rejection region from the non-rejection region in hypothesis testing. It is compared to the test statistic to determine whether to reject or fail to reject the null hypothesis.Expected Successes and Failures
: Expected successes and failures are calculated probabilities of success and failure based on some underlying model or assumption.Large Counts Condition
: The large counts condition, also known as the "success-failure" condition, is used when applying certain statistical methods to categorical data. It states that for these methods to be valid, both the number of successes and failures must be at least 10.Margin of Error
: The margin of error is a measure of the uncertainty or variability in survey results. It represents the range within which the true population parameter is likely to fall.p-value (in the context of a given proportion)
: The p-value is the probability of obtaining a sample proportion as extreme or more extreme than the observed proportion, assuming that the null hypothesis is true.Random Sample
: A random sample is a subset of individuals selected from a larger population in such a way that every individual has an equal chance of being chosen. It helps to ensure that the sample is representative of the population.Sample Data
: Sample data refers to a subset of observations or measurements taken from a larger population. It is used to make inferences and draw conclusions about the entire population.Sampling Distribution
: A sampling distribution refers to the distribution of a statistic (such as mean, proportion, or difference) calculated from multiple random samples taken from the same population. It provides information about how sample statistics vary from sample to sample.Statistical Claim
: A statistical claim is a statement or hypothesis about a population parameter that can be tested using statistical methods.True Population Proportion
: The true population proportion refers to the actual proportion or percentage of a specific characteristic or attribute within an entire population. It is often estimated using sample data.z*
: In statistics, z* refers to the standardized score obtained from the standard normal distribution. It represents the number of standard deviations a data point is away from the mean.
Resources
© 2024 Fiveable Inc. All rights reserved.
AP® and SAT® are trademarks registered by the College Board, which is not affiliated with, and does not endorse this website.
