SAT Math: Guide to Quadratic Equations & Radicals

tl;dr: Quadratic equations and radicals in the SAT Math section  include topics such as using the quadratic formula, factoring polynomials, interpreting graphs and functions, and determining roots and square roots. The practice problems below will test your ability to rewrite expressions, add/subtract/multiply polynomial expressions, utilize the distributive property, use the quadratic formula, and apply the principles of quadratic equations & factoring.

These questions (both with-calculator and no-calculator) test on four major content areas: the Heart of Algebra, Problem Solving, Data Analysis, and the Passport to Advanced Math.

How long is the SAT Math section?

The SAT Math section contains approximately 60 questions, separated into:

• No-Calculator Section

• 15 Multiple Choice
• 5 Grid-Ins
• 25 Minutes

• Calculator Section

• 30 Multiple Choice
• 8 Grid-Ins
• 55 Minutes

• Total Time: 80 Minutes

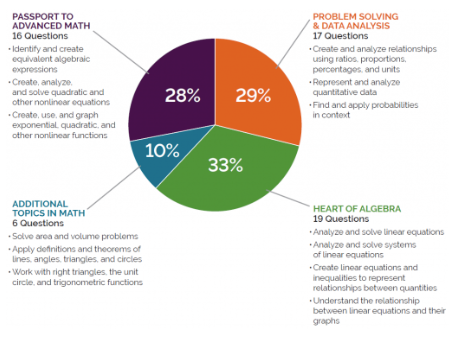
Quadratic Equations and Radicals fall under the Passport to Advanced Math section. Topics pertaining to “Quadratic Equations & Radicals”:

• Quadratic Equations & Expressions
• Function Interpretation
• Graph Interpretation
• Roots
• Square Roots
• Squaring

What you will have to be able to do:

• Use the quadratic formula
• Factor and multiply polynomial expressions (word problems, etc.)
• Interpret graphs and functions to write quadratic expressions
• Determine the roots & square roots of various functions and equations

SAT Math Section Rules

Directions for the Non-Calculator Section

• The use of a calculator is not permitted.
• All variables and expressions used represent real numbers unless otherwise indicated.
• Figures provided in this test are drawn to scale unless otherwise indicated.
• All figures lie in a plane unless otherwise indicated.

SAT Math Quadratics Practice Problems

Non-calculator practice problems:

1. Which of the following is equivalent to 16s4 - 4t2?

(a) 4(s2 − t )(4s2 + t )

(b) 4(4s2 - t)(s2 - t)

(c) 4(2s2 − t )(2s2 + t )

(d) (8s2 − 2t )(8s2 + 2t )

The correct answer is C.

Solution & Advice:

• Recognize something familiar about this problem? It’s a difference of squares!

• This classic quadratic pattern draws from x2 - y2, which can be factored into (x-y)(x+y). Recognizing these patterns can help you answer questions quickly and help you set a steady pace on the exam.

• How does this question demonstrate an understanding of the difference of squares (more specifically, the difference of perfect squares)?

• Let’s break it down!

⇒ 16s4 - 4t2

⇒ = (4s2)2 - (2t)2 (difference of perfect squares)

⇒ =  (4s2 − 2t ) (4s2 + 2t) (an extension of (x+y)(x-y))

⇒ = 2(2s2 − t )(2)(2s2 + t ) (factor out a 2!)

⇒ = 4(2s2 − t ) (2s2 + t ) (Multiply your factors of 2 = 4!)

⇒ This leads us to Option C!

• This question tests your ability to rewrite expressions, and add/subtract/multiply polynomial expressions.

Feeling good? Let’s try another one!

2. (x2 + bx − 2) (x + 3) = x3 + 6x2 + 7x − 6

In the equation above, b is a constant. If the equation is true for all values of x, what is the value of b?

(a) 2

(b) 3

(c) 7

(d) 9

The correct answer is B.

Solution & Advice:

• This question tests your ability to utilize the distributive property. Let’s expand this equation!

• The key to this question is to convert one side of the equation to match the other side in order to compare like terms and determine the value of b!
• Left Hand Side = (x2 + bx − 2)(x + 3)

⇒ = (x3 + bx2 − 2x ) + (3x2 + 3bx − 6)

⇒ = x3 + (3 + b) x2 + (3b − 2)(x − 6)

• Right Hand Side = x3 + 6x2 + 7x − 6

⇒ Now that all like terms have been combined and expanded on the Left Hand Side, we can compare them to the Right Hand Side! It’s visible that (3+b) = 6, therefore b = 3

• This question tests your ability to rewrite expressions, and add/subtract/multiply polynomial expressions.

Time for Question 3! Looking good!

3) What are the solutions to the equation x2 − 3 = x?

(a) (−1 ± √11)/2

(b) (−1 ± _√13)/2

(c) (1 ± √11)/2

(d) (1 ± √13)/2

The correct answer is D.

Solution & Advice:

• This question draws on your ability to use the quadratic formula and completing the square. How so? Let’s find out!

• First, subtract x from each side to convert the equation into standard form

• Standard Form: ax2 + bc + c

• Now, you’re left with x2 - x - 3 = 0
• Comparing this to the standard form above, you can note that a = 1, b = -1, and c = -3!
• You can plug these values into the quadratic formula, and simplify to get Choice D!

• This question tests your ability to utilize radicals, and apply the quadratic formula.

Time for Question 4! How are you feeling?  

(4) x − 12 = √(x + 44)

What are all possible solutions to the given equation?

(a) 5

(b) 20

(c) −5 and 20

(d) 5 and 20

The correct answer is B.

Solution & Advice:

• This question draws on your ability to use the radicals and use the quadratic equation. Let’s break it down:  

• x − 12 = √(x + 44)
• First, square both sides of the equation!

⇒ = (x - 12)2 = (x + 44)

⇒ = x2 - 24x + 144 = x + 144

⇒ x2 - 25x + 100 = 0

⇒ (x-5)(x-20)

Seems like 5 and 20 both satisfy our requirements! But wait, there’s one more check! We need to plug both 5 and 20 back into the original equation to ensure they work.

After plugging both 5 and 20 in, only 20 works, therefore Choice B is correct!

• This question tests your ability to utilize radicals, and apply the principles of quadratic equations & factoring.

Closing

Congratulations! You’ve made it to the end of this prep activity 🙌. You learned about “SAT Math - Quadratic Equations & Radicals”. You should have a better understanding of the Math sections for the SAT© , topic highlights, what you will have to be able to do in order to succeed, as well as have seen some practice questions that put the concepts in action. Good luck studying for the SAT Math section 👏!

Need more resources? Check out our complete SAT Math Study Guide w/ Practice Problems.